Note
Go to the end to download the full example code
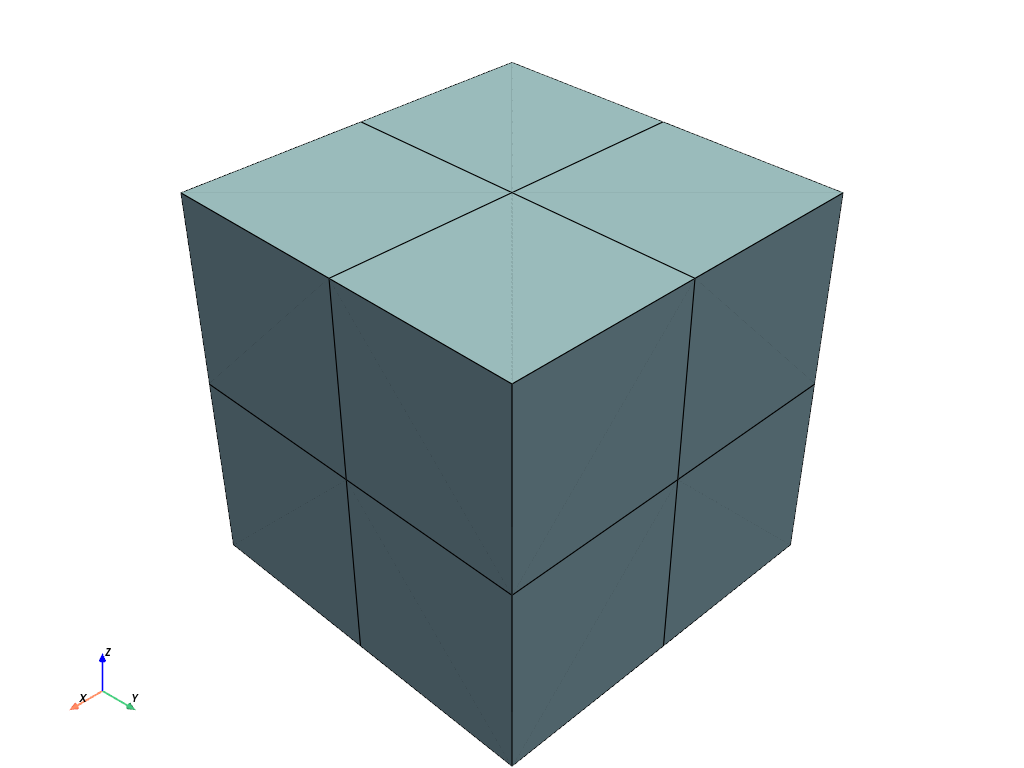
Creating an Unstructured Grid#
Create an irregular, unstructured grid from NumPy arrays.
import numpy as np
import pyvista as pv
from pyvista import CellType
An unstructured grid can be created directly from NumPy arrays. This is useful when creating a grid from scratch or copying it from another format. See vtkUnstructuredGrid for available cell types and their descriptions.
# Contains information on the points composing each cell.
# Each cell begins with the number of points in the cell and then the points
# composing the cell
cells = np.array([8, 0, 1, 2, 3, 4, 5, 6, 7, 8, 8, 9, 10, 11, 12, 13, 14, 15])
# cell type array. Contains the cell type of each cell
cell_type = np.array([CellType.HEXAHEDRON, CellType.HEXAHEDRON])
# in this example, each cell uses separate points
cell1 = np.array(
[
[0, 0, 0],
[1, 0, 0],
[1, 1, 0],
[0, 1, 0],
[0, 0, 1],
[1, 0, 1],
[1, 1, 1],
[0, 1, 1],
]
)
cell2 = np.array(
[
[0, 0, 2],
[1, 0, 2],
[1, 1, 2],
[0, 1, 2],
[0, 0, 3],
[1, 0, 3],
[1, 1, 3],
[0, 1, 3],
]
)
# points of the cell array
points = np.vstack((cell1, cell2)).astype(float)
# create the unstructured grid directly from the numpy arrays
grid = pv.UnstructuredGrid(cells, cell_type, points)
# For cells of fixed sizes (like the mentioned Hexahedra), it is also possible to use the
# simplified dictionary interface. This automatically calculates the cell array.
# Note that for mixing with additional cell types, just the appropriate key needs to be
# added to the dictionary.
cells_hex = np.arange(16).reshape([2, 8])
# = np.array([[0, 1, 2, 3, 4, 5, 6, 7], [8, 9, 10, 11, 12, 13, 14, 15]])
grid = pv.UnstructuredGrid({CellType.HEXAHEDRON: cells_hex}, points)
# plot the grid (and suppress the camera position output)
_ = grid.plot(show_edges=True)
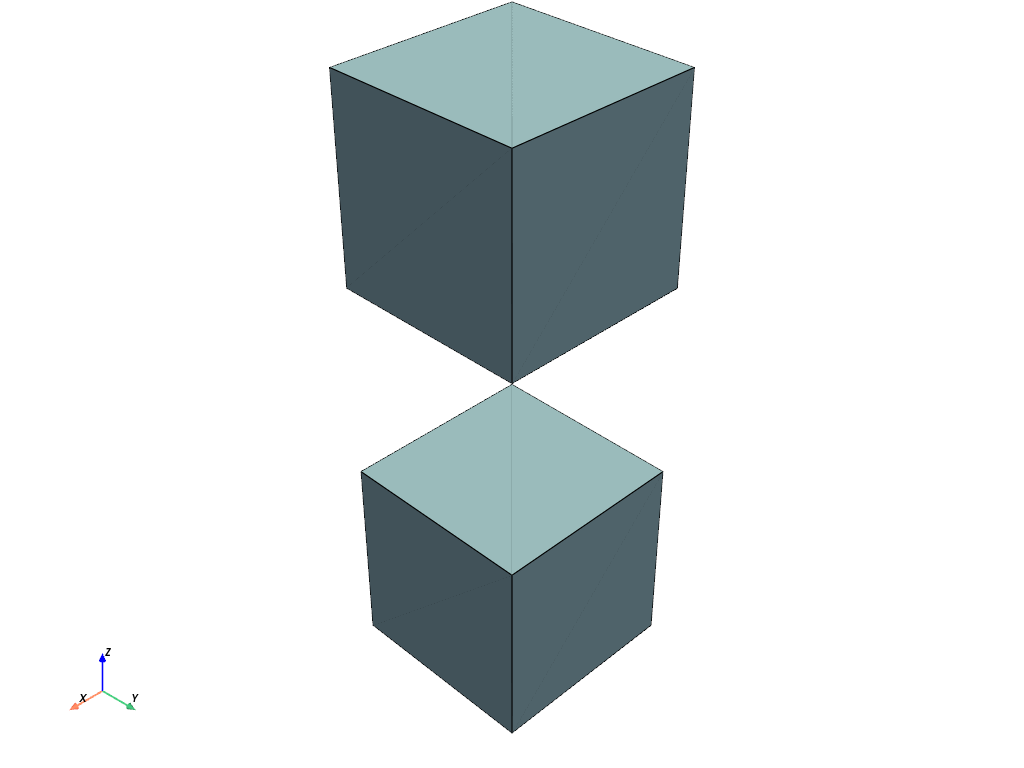
Tetrahedral Grid#
Here is how we can create an unstructured tetrahedral grid.
# There are 10 cells here, each cell is [4, INDEX0, INDEX1, INDEX2, INDEX3]
# where INDEX is one of the corners of the tetrahedron.
#
# Note that the array does not need to be shaped like this, we could have a
# flat array, but it's easier to make out the structure of the array this way.
cells = np.array(
[
[4, 6, 5, 8, 7],
[4, 7, 3, 8, 9],
[4, 7, 3, 1, 5],
[4, 9, 3, 1, 7],
[4, 2, 6, 5, 8],
[4, 2, 6, 0, 4],
[4, 6, 2, 0, 8],
[4, 5, 2, 8, 3],
[4, 5, 3, 8, 7],
[4, 2, 6, 4, 5],
]
)
celltypes = np.full(10, fill_value=CellType.TETRA, dtype=np.uint8)
# These are the 10 points. The number of cells does not need to match the
# number of points, they just happen to in this example
points = np.array(
[
[-0.0, 0.0, -0.5],
[0.0, 0.0, 0.5],
[-0.43, 0.0, -0.25],
[-0.43, 0.0, 0.25],
[-0.0, 0.43, -0.25],
[0.0, 0.43, 0.25],
[0.43, 0.0, -0.25],
[0.43, 0.0, 0.25],
[0.0, -0.43, -0.25],
[0.0, -0.43, 0.25],
]
)
# Create and plot the unstructured grid
grid = pv.UnstructuredGrid(cells, celltypes, points)
grid.plot(show_edges=True)
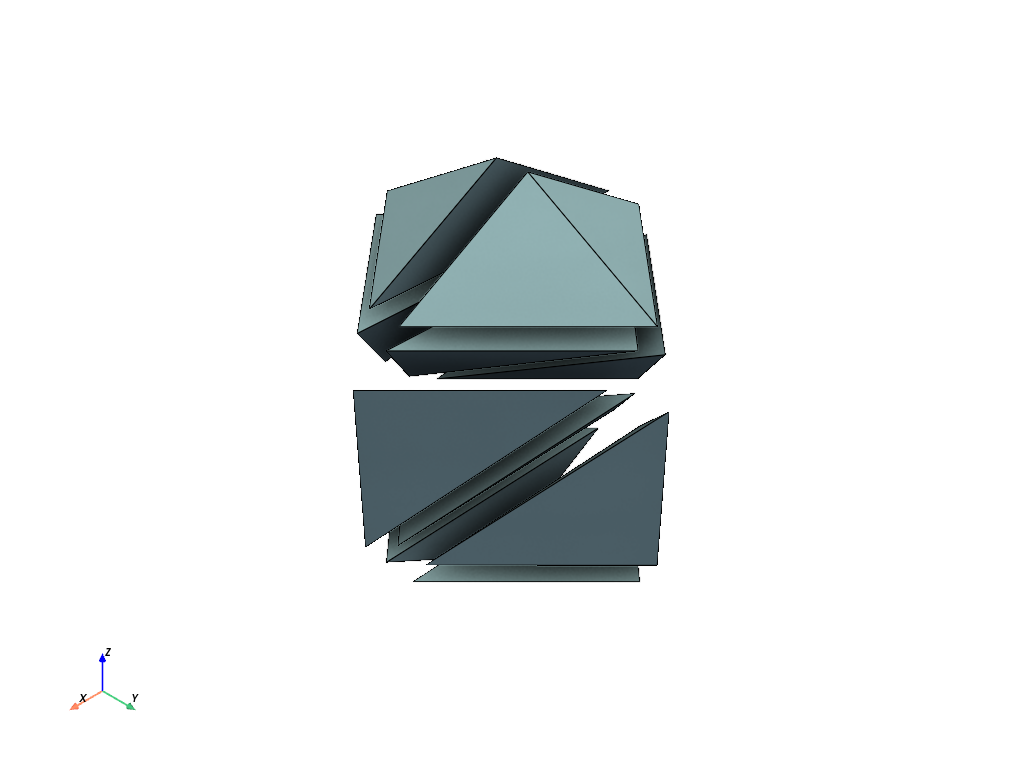
For fun, let’s separate all the cells and plot out the individual cells. Shift them a little bit from the center to create an “exploded view”.
split_cells = grid.explode(0.5)
split_cells.plot(show_edges=True, ssao=True)
Total running time of the script: (0 minutes 1.470 seconds)